
We note that Young has provided a schematic overview of atomic volumes at selected higher pressures obtained by an amalgamation of experiment and calculation on condensed phases. Atomic volumes can, and have been, measured as a function of pressure using a variety of experimental techniques (see, e.g., ref. We can, therefore, in principle, relate the volume V of eqn (2) to an average atomic volume. How does eqn (2) thus relate to atomic radii? The challenge of estimating atomic radii at high pressure Pressure is a macroscopic observable, defined in terms of an ensemble of atoms. We refer to eqn (2) as an “Experimental Quantum Chemistry” partitioning 50 because it is, in principle, possible to estimate all of its terms directly or indirectly from a combination of thermal measurements, photoelectron spectroscopy, X-ray diffraction structure determination, and, for the Δ( pV)-term, equations of state. The relationship between enthalpy, electronegativity, nuclear geometry, electron interactions, pressure, and volume provided by eqn (2) is exact within the Born–Oppenheimer approximation. Where Δ( pV) describes changes in volume V and pressure p. Specifically, for compression at T → 0 K, we can write:
#ATOMIC SIZE TREND FREE#
Where Δ E is the change in total energy over a chemical or physical transformation, n is the total number of electrons, Δ is the change in electronegativity (here defined as the average electron binding energy), while Δ E ee and Δ E NN are changes in the electrostatic repulsion between electrons (under the influence of exchange and correlation effects) and nuclei, respectively.Įqn (1) can be recast to also partition relative enthalpies or free energies. 29,50 This definition of electronegativity establishes a connection with the total energy of a system through an energy decomposition analysis: 51 The scale of electronegativity used here is inspired by the work of Allen 26 and is defined as the average electron binding energy as T → 0 K. 48 In this work, we rely on two revised scales of atomic vdW radii 3 and electronegativity 29 which have been extended to high pressure conditions (0–300 GPa).
have calculated the critical diameter at which spherical confinement causes ionization of some atoms. 45 and Chattaraj and co-workers 46,47 have, for example, relied on conceptual density functional theory to evaluate the electronegativity for a selection of atoms compressed by impenetrable spherical cavities. There exist different frameworks through which electronegativity might be related to radii under compression. The means by which we change the size of individual atoms is through physical compression. In this work we take a different perspective and instead consider how electronegativity changes as the sizes of individual atoms are modified. Thus far, relationships between atomic radii and electronegativity have mostly been sought under ambient conditions and have been rationalized by comparing different atoms. Pitzer pointed out the periodic behavior in the two atomic properties long ago 30 and many others have relied on different definitions of atomic radii (usually covalent radii) and electrostatic relationships to define scales of electronegativity.
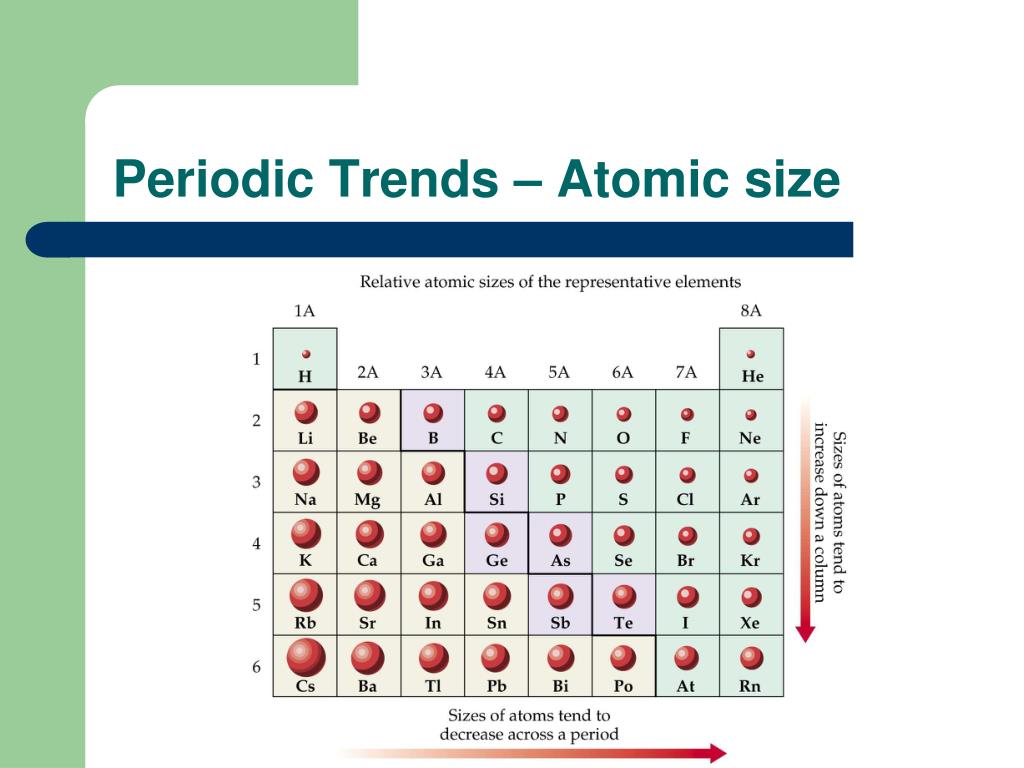
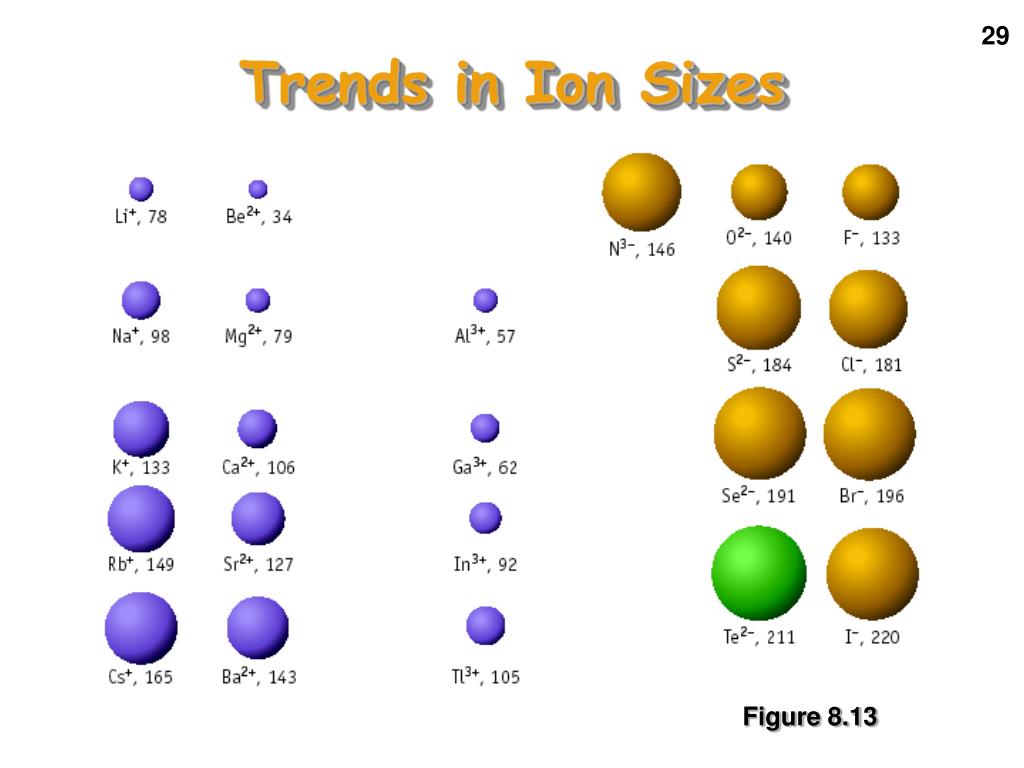
The closer the electrons are to the nucleus, the more tightly they are bound, thus increasing the electronegativity of the atom. 22–29 and references therein).Ī relationship is intuitively expected between electronegativity and radius: the size of an atom is determined by the distribution of electrons around its nucleus. 3,18–21 Electronegativity is a similarly well-studied concept that can be defined in many ways (see, e.g., ref. 7–9 Today, a variety of definitions of atomic radii with well-known uses exists, including, e.g., ionic, 10–12 covalent, 6,13–17 and vdW radii. One early motivation for attaining atomic and ionic sizes was to help understand X-ray diffraction patterns in terms of crystal structures, 5,6 another to provide a rationalization for metallization. 1,2 The history of quantifying the sizes of atoms under ambient conditions includes a large body of work, extending over the last one and a half-centuries (for a non-exhaustive summary of this history see ref.

Introduction Atomic radii and electronegativity are often quintessential for how chemistry is rationalized. Here, we show how compression can reveal a long sought-after connection between two central chemical concepts – van-der-Waals (vdW) radii and electronegativity – and how these relate to the driving forces behind chemical and physical transformations. Trends in atomic properties are well-established tools for guiding the analysis and discovery of materials.


 0 kommentar(er)
0 kommentar(er)
